170 D1.03: Examples 6–9
Example 6
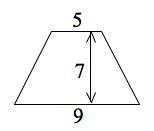
Find the area of this trapezoid, where the numbers represent feet.
Show Answer
The formula is [latex]A=\frac{1}{2}h\left({{b}_{1}}+{{b}_{2}}\right)[/latex]where h is the height, [latex]{{b}_{1}}[/latex] and [latex]{{b}_{2}}[/latex] are the lengths of the two bases, and A is the area. Notice that the subscripts are used because both these sides are called bases, so b is a reasonable letter to use, but there are two different ones. We use subscripts on the b’s to distinguish between the two different bases.
Example 7
Find the volume of a sphere with radius 3 inches. The formula is [latex]V=\frac{4}{3}\pi{{r}^{3}}[/latex].
Show Answer
[latex]V=\frac{4}{3}\pi{{r}^{3}}=\frac{4}{3}\pi{{\left(3\right)}^{3}}=\frac{4}{3}\pi\cdot27=113.097[/latex].
So the volume of this sphere is 113.097 cubic inches.
Example 8
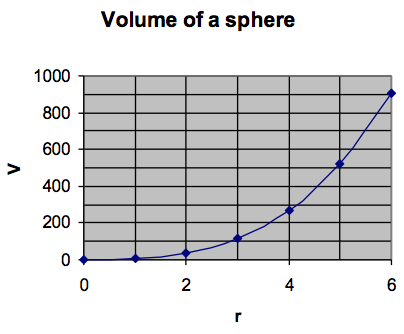
Graph the formula [latex]V=\frac{4}{3}\pi{{r}^{3}}[/latex] for the values of r from 0 to 6 feet and use the graph to approximate what radius will give a volume of 400 cubic feet.
Show Answer
Notice that r is the input value and V is the output value.
| r | V |
|---|---|
| 0 | 0 |
| 1 | 4.188787 |
| 2 | 33.51029 |
| 3 | 113.0972 |
| 4 | 268.0823 |
| 5 | 523.5983 |
| 6 | 904.7779 |
Using the graph, we follow the line for 400 on the vertical axis across to the graph and then down to see the corresponding r value, which is approximately [latex]r=4.5[/latex] feet. We can check this by plugging in and finding that [latex]V=\frac{4}{3}\pi{{(4.5)}^{3}}=381.7[/latex]
If we wanted to get a better estimate, we would try a somewhat higher value for r.
Example 9
Consider the problem of Example 8. Use graphical and numerical methods to find a value for the radius that will give a volume of 400 cubic feet, correct to within 10 cubic feet.
Show Answer
The graph tells us that a good estimate for the radius is 4.5 feet. Next we check that by plugging in and finding that [latex]V=\frac{4}{3}\pi{{(4.5)}^{3}}=381.7[/latex]cubic feet. That’s a bit too low, so let’s try a slightly larger value for the radius. Let’s try 4.6 feet. [latex]V=\frac{4}{3}\pi{{(4.6)}^{3}}=407.72[/latex] cubic feet. That’s an adequate answer, according to the tolerance level stated in the problem. Of course, if we wanted a more accurate answer, we could continue to work numerically by taking a value for the radius just a bit smaller than 4.6 feet and finding the volume for that.
Perhaps you have noticed that you could use algebra (involving cube roots) instead of the graph on the problem of Examples 8 and 9 to obtain a quite exact answer for the radius needed to obtain a volume of 400 cubic feet. And that’s a fine thing to do. But, in this course, we are practicing this technique that will allow you to solve problems of this kind even if the formula is so complicated that the algebra needed to “invert” the formula is harder than anything you’ve learned in your algebra courses. In fact, some formulas are so complicated that even mathematicians find these values in just the same way you’re learning here. Using graphs in this way is a very powerful and important tool in working on technical problems!