134 C1.05: Interval Part 1

Section 4. Write an interval of actual values that are consistent with a rounded value.

We can represent all possible real numbers on a number line. We can only label a few of the points because if we tried to label more, the picture would become confusing. But you should think of being able to graph on a number line any particular number you are given. (When doing these problems, estimate distances rather than trying to measure them exactly.)

Example 1. Draw a number line that includes the values from 0 to 3. On that number line, label the points corresponding to the three numbers 0.1, 1.56, and 2.478.

Example 2. Draw a number line that includes the values from 2.0 to 3.0 and label the points corresponding to these numbers: 2.0, 2.1, 2.2, 2.3, 2.4, 2.5, 2.6, 2.7, 2.8, 2.9, and 3.0.

Example 3. Draw a number line that includes the values from 2.2 to 2.4, and label 2.2, 2.3, and 2.4.
(We will now take some numbers in that range with more decimal places, graph them on the number line, and then consider how to round each of those numbers to the nearest tenth.)

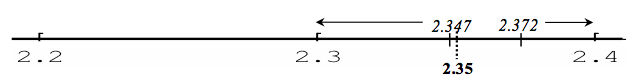
Consider the two numbers 2.347 and 2.372. Place each of them reasonably correctly on the number line.

Round each of these numbers to the nearest tenth and use an arrow to show which number it is rounded to.

What is the cut-off value that separates the numbers that are rounded to 2.3 and those that are rounded to 2.4?   Indicate that value on the number line and label it.

Notice that the cut-off value separating the numbers that are rounded to 2.3 and those rounded to 2.4 is 2.35, which is halfway between 2.3 and 2.4.

Example 4.   We will draw the interval of actual values that are consistent with the rounded value of 2.3.

Procedure:  

• Think of numbers near 2.3 that have the same precision. Since the precision of 2.3 is one-tenth, then other nearby numbers are 2.1, 2.2, 2.3, 2.4, 2.5, etc.
• Choose numbers with the same precision—the given 2.3 and the ones on either side, which are 2.2 and 2.4. Put them on a number line.
• What is the cut-off value that separates the number that are rounded to 2.2 and the numbers that are rounded to 2.3? Indicate that value on the number line.
• To label that value, it is easiest to go back and put an extra zero on all the other values, so you have 2.20, 2.30, and 2.40. That helps clarify that the value half-way between 2.20 and 2.30 is 2.25. Label it.
• What is the cut-off value that separates the numbers that are rounded to 2.3 and the numbers that are rounded to 2.4? Indicate that value on the number line and label it.
• Indicate the interval of values that are rounded to 2.3.